how to find y intercept of a rational function
Polynomial and Rational Functions
Rational Functions
Learning Objectives
In this section, you will:
- Employ arrow notation.
- Solve applied problems involving rational functions.
- Discover the domains of rational functions.
- Place vertical asymptotes.
- Place horizontal asymptotes.
- Graph rational functions.
Suppose nosotros know that the cost of making a product is dependent on the number of items,![]() produced. This is given by the equation
produced. This is given by the equation![]() If nosotros want to know the boilerplate toll for producing
If nosotros want to know the boilerplate toll for producing![]() items, we would dissever the price part by the number of items,
items, we would dissever the price part by the number of items,![]()
The average cost function, which yields the average price per item for![]() items produced, is
items produced, is
![]()
Many other application issues require finding an boilerplate value in a like manner, giving us variables in the denominator. Written without a variable in the denominator, this role volition contain a negative integer power.
In the last few sections, we have worked with polynomial functions, which are functions with non-negative integers for exponents. In this section, we explore rational functions, which take variables in the denominator.
Using Pointer Annotation
We take seen the graphs of the bones reciprocal function and the squared reciprocal function from our written report of toolkit functions. Examine these graphs, equally shown in (Figure), and discover some of their features.

Several things are credible if we examine the graph of![]()
- On the left branch of the graph, the curve approaches the x-axis

- Every bit the graph approaches
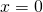 from the left, the curve drops, simply every bit nosotros approach zero from the right, the curve rises.
from the left, the curve drops, simply every bit nosotros approach zero from the right, the curve rises. - Finally, on the right branch of the graph, the curves approaches the 10-axis
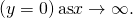
To summarize, nosotros use arrow notation to show that![]() or
or![]() is budgeted a particular value. Meet (Figure).
is budgeted a particular value. Meet (Figure).
| Symbol | Meaning |
|---|---|
| | |
| | |
| | |
| | |
| | the output approaches infinity (the output increases without bound) |
| | the output approaches negative infinity (the output decreases without bound) |
| | the output approaches |
Local Behavior of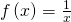
Let's begin by looking at the reciprocal function,![]() We cannot split up by naught, which means the part is undefined at
We cannot split up by naught, which means the part is undefined at![]() then goose egg is not in the domain. As the input values arroyo zero from the left side (becoming very small, negative values), the role values decrease without bound (in other words, they approach negative infinity). We tin can see this beliefs in (Figure).
then goose egg is not in the domain. As the input values arroyo zero from the left side (becoming very small, negative values), the role values decrease without bound (in other words, they approach negative infinity). We tin can see this beliefs in (Figure).
| | –0.1 | –0.01 | –0.001 | –0.0001 |
| | –x | –100 | –m | –10,000 |
We write in arrow notation
![]()
Every bit the input values approach aught from the right side (becoming very small, positive values), the function values increase without spring (approaching infinity). We can see this behavior in (Effigy).
| | 0.i | 0.01 | 0.001 | 0.0001 |
| | 10 | 100 | 1000 | 10,000 |
We write in arrow annotation
![]()
See (Figure).

This behavior creates a vertical asymptote, which is a vertical line that the graph approaches but never crosses. In this case, the graph is approaching the vertical line![]() as the input becomes close to cipher. See (Effigy).
as the input becomes close to cipher. See (Effigy).

Vertical Asymptote
A vertical asymptote of a graph is a vertical line![]() where the graph tends toward positive or negative infinity as the inputs approach
where the graph tends toward positive or negative infinity as the inputs approach![]() We write
We write
![]()
End Behavior of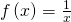
As the values of![]() arroyo infinity, the function values approach 0. Equally the values of
arroyo infinity, the function values approach 0. Equally the values of![]() arroyo negative infinity, the function values arroyo 0. Come across (Figure). Symbolically, using arrow annotation
arroyo negative infinity, the function values arroyo 0. Come across (Figure). Symbolically, using arrow annotation
![]()

Based on this overall beliefs and the graph, we can see that the function approaches 0 but never actually reaches 0; information technology seems to level off equally the inputs become large. This beliefs creates a horizontal asymptote, a horizontal line that the graph approaches as the input increases or decreases without bound. In this case, the graph is budgeted the horizontal line![]() See (Figure).
See (Figure).

Horizontal Asymptote
A horizontal asymptote of a graph is a horizontal line![]() where the graph approaches the line equally the inputs increase or decrease without spring. Nosotros write
where the graph approaches the line equally the inputs increase or decrease without spring. Nosotros write
![]()
Using Arrow Notation
Utilise arrow notation to describe the stop behavior and local beliefs of the function graphed in (Figure).

[reveal-answer q="fs-id1165137870943″]Show Solution[/reveal-answer]
[hidden-reply a="fs-id1165137870943″]
Detect that the graph is showing a vertical asymptote at![]() which tells us that the function is undefined at
which tells us that the function is undefined at![]()
![]()
And as the inputs subtract without bound, the graph appears to be leveling off at output values of 4, indicating a horizontal asymptote at![]() As the inputs increase without spring, the graph levels off at 4.
As the inputs increase without spring, the graph levels off at 4.
![]() [/hidden-answer]
[/hidden-answer]
Try Information technology
Employ pointer note to describe the cease beliefs and local behavior for the reciprocal squared function.
[reveal-reply q="fs-id1165135541751″]Bear witness Solution[/reveal-answer]
[hidden-reply a="fs-id1165135541751″]
End behavior: every bit![]() Local behavior: as
Local behavior: as![]() (there are no x– or y-intercepts)
(there are no x– or y-intercepts)
[/subconscious-respond]
Using Transformations to Graph a Rational Function
Sketch a graph of the reciprocal role shifted two units to the left and upwardly iii units. Identify the horizontal and vertical asymptotes of the graph, if whatever.
[reveal-answer q="fs-id1165137911752″]Bear witness Solution[/reveal-reply]
[hidden-answer a="fs-id1165137911752″]
Shifting the graph left 2 and up iii would outcome in the function
![]()
or equivalently, by giving the terms a common denominator,
![]()
The graph of the shifted function is displayed in (Effigy).

Notice that this function is undefined at![]() and the graph also is showing a vertical asymptote at
and the graph also is showing a vertical asymptote at![]()
![]()
As the inputs increase and subtract without bound, the graph appears to be leveling off at output values of 3, indicating a horizontal asymptote at![]()
![]() [/subconscious-respond]
[/subconscious-respond]
Analysis
Notice that horizontal and vertical asymptotes are shifted left 2 and up iii along with the function.
Try It
Sketch the graph, and find the horizontal and vertical asymptotes of the reciprocal squared function that has been shifted right 3 units and down 4 units.
[reveal-answer q="fs-id1165137812954″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id1165137812954″]

The role and the asymptotes are shifted 3 units right and 4 units downwards. As![]() and equally
and equally![]()
The function is![]()
[/hidden-respond]
Solving Applied Problems Involving Rational Functions
In (Figure), we shifted a toolkit function in a way that resulted in the function![]() This is an example of a rational role. A rational function is a office that tin be written as the quotient of two polynomial functions. Many real-world problems require the states to notice the ratio of ii polynomial functions. Issues involving rates and concentrations often involve rational functions.
This is an example of a rational role. A rational function is a office that tin be written as the quotient of two polynomial functions. Many real-world problems require the states to notice the ratio of ii polynomial functions. Issues involving rates and concentrations often involve rational functions.
Rational Part
A rational role is a role that tin can be written as the quotient of two polynomial functions![]()
![]()
Solving an Applied Problem Involving a Rational Office
A big mixing tank currently contains 100 gallons of water into which 5 pounds of saccharide have been mixed. A tap volition open pouring 10 gallons per infinitesimal of water into the tank at the same time sugar is poured into the tank at a rate of 1 pound per infinitesimal. Discover the ratio of sugar to h2o, in pounds per gallon in the tank after 12 minutes. Is that a greater ratio of sugar to water, in pounds per gallon than at the beginning?
[reveal-respond q="fs-id1165137526522″]Evidence Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137526522″]
Let![]() be the number of minutes since the tap opened. Since the h2o increases at 10 gallons per minute, and the sugar increases at 1 pound per infinitesimal, these are constant rates of modify. This tells us the amount of water in the tank is changing linearly, as is the amount of sugar in the tank. Nosotros can write an equation independently for each:
be the number of minutes since the tap opened. Since the h2o increases at 10 gallons per minute, and the sugar increases at 1 pound per infinitesimal, these are constant rates of modify. This tells us the amount of water in the tank is changing linearly, as is the amount of sugar in the tank. Nosotros can write an equation independently for each:
![]()
The ratio of sugar to water, in pounds per gallon,![]() , will exist the ratio of pounds of sugar to gallons of water
, will exist the ratio of pounds of sugar to gallons of water
![]()
The ratio of sugar to water, in pounds per gallon after 12 minutes is given by evaluating![]() at
at![]()
![]()
This means the ratio of sugar to h2o, in pounds per gallon is 17 pounds of carbohydrate to 220 gallons of water.
At the beginning, the ratio of sugar to h2o, in pounds per gallon is
![]()
Since![]() the ratio of sugar to water, in pounds per gallon is greater after 12 minutes than at the beginning.[/subconscious-reply]
the ratio of sugar to water, in pounds per gallon is greater after 12 minutes than at the beginning.[/subconscious-reply]
Try It
In that location are i,200 freshmen and i,500 sophomores at a prep rally at noon. Later on 12 p.1000., twenty freshmen arrive at the rally every 5 minutes while 15 sophomores leave the rally. Find the ratio of freshmen to sophomores at 1 p.m.
[reveal-answer q="fs-id1165137644596″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137644596″]
![]()
[/hidden-answer]
Finding the Domains of Rational Functions
A vertical asymptote represents a value at which a rational part is undefined, and then that value is not in the domain of the function. A reciprocal office cannot have values in its domain that crusade the denominator to equal zero. In general, to find the domain of a rational role, we need to determine which inputs would crusade sectionalization past nothing.
Domain of a Rational Function
The domain of a rational part includes all real numbers except those that cause the denominator to equal null.
How To
Given a rational function, observe the domain.
- Set the denominator equal to nix.
- Solve to find the x-values that cause the denominator to equal zero.
- The domain is all real numbers except those found in Step 2.
Finding the Domain of a Rational Function
Find the domain of![]()
[reveal-answer q="fs-id1165137656082″]Show Solution[/reveal-respond]
[hidden-reply a="fs-id1165137656082″]
Begin past setting the denominator equal to zero and solving.
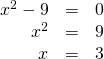
The denominator is equal to zip when![]() The domain of the function is all real numbers except
The domain of the function is all real numbers except![]()
[/hidden-respond]
Analysis
A graph of this function, as shown in (Figure), confirms that the function is non defined when![]()

There is a vertical asymptote at![]() and a pigsty in the graph at
and a pigsty in the graph at![]() Nosotros volition discuss these types of holes in greater detail later in this section.
Nosotros volition discuss these types of holes in greater detail later in this section.
Effort It
Observe the domain of![]()
[reveal-answer q="fs-id1165137474960″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137474960″]
The domain is all real numbers except![]() and
and![]()
[/subconscious-answer]
Identifying Vertical Asymptotes of Rational Functions
Past looking at the graph of a rational function, nosotros tin can investigate its local behavior and easily see whether in that location are asymptotes. We may even be able to guess their location. Even without the graph, yet, nosotros can even so determine whether a given rational function has any asymptotes, and calculate their location.
Vertical Asymptotes
The vertical asymptotes of a rational role may be found by examining the factors of the denominator that are not mutual to the factors in the numerator. Vertical asymptotes occur at the zeros of such factors.
Given a rational role, place whatsoever vertical asymptotes of its graph.
- Gene the numerator and denominator.
- Annotation whatever restrictions in the domain of the function.
- Reduce the expression past canceling mutual factors in the numerator and the denominator.
- Note any values that cause the denominator to be zero in this simplified version. These are where the vertical asymptotes occur.
- Note any restrictions in the domain where asymptotes do non occur. These are removable discontinuities, or "holes."
Identifying Vertical Asymptotes
Discover the vertical asymptotes of the graph of![]()
[reveal-answer q="fs-id1165137389408″]Show Solution[/reveal-reply]
[hidden-reply a="fs-id1165137389408″]
Commencement, factor the numerator and denominator.
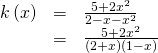
To find the vertical asymptotes, we decide where this office will be undefined by setting the denominator equal to naught:
![]()
Neither![]() nor
nor![]() are zeros of the numerator, so the ii values indicate two vertical asymptotes. The graph in (Figure) confirms the location of the ii vertical asymptotes.
are zeros of the numerator, so the ii values indicate two vertical asymptotes. The graph in (Figure) confirms the location of the ii vertical asymptotes.

[/hidden-respond]
Removable Discontinuities
Occasionally, a graph will contain a hole: a single point where the graph is not defined, indicated by an open circumvolve. We call such a hole a removable aperture.
For instance, the role![]() may be re-written by factoring the numerator and the denominator.
may be re-written by factoring the numerator and the denominator.
![]()
Notice that![]() is a common gene to the numerator and the denominator. The zip of this factor,
is a common gene to the numerator and the denominator. The zip of this factor,![]() is the location of the removable discontinuity. Notice also that
is the location of the removable discontinuity. Notice also that![]() is non a cistron in both the numerator and denominator. The zero of this factor,
is non a cistron in both the numerator and denominator. The zero of this factor,![]() is the vertical asymptote. See (Figure). [Notation that removable discontinuities may not exist visible when we use a graphing calculator, depending upon the window selected.]
is the vertical asymptote. See (Figure). [Notation that removable discontinuities may not exist visible when we use a graphing calculator, depending upon the window selected.]

Removable Discontinuities of Rational Functions
A removable discontinuity occurs in the graph of a rational part at![]() if
if![]() is a nil for a factor in the denominator that is common with a factor in the numerator. We cistron the numerator and denominator and check for common factors. If nosotros find any, we gear up the mutual gene equal to 0 and solve. This is the location of the removable discontinuity. This is true if the multiplicity of this factor is greater than or equal to that in the denominator. If the multiplicity of this cistron is greater in the denominator, then in that location is still an asymptote at that value.
is a nil for a factor in the denominator that is common with a factor in the numerator. We cistron the numerator and denominator and check for common factors. If nosotros find any, we gear up the mutual gene equal to 0 and solve. This is the location of the removable discontinuity. This is true if the multiplicity of this factor is greater than or equal to that in the denominator. If the multiplicity of this cistron is greater in the denominator, then in that location is still an asymptote at that value.
Identifying Vertical Asymptotes and Removable Discontinuities for a Graph
Observe the vertical asymptotes and removable discontinuities of the graph of![]()
[reveal-answer q="fs-id1165137425735″]Bear witness Solution[/reveal-answer]
[hidden-reply a="fs-id1165137425735″]
Factor the numerator and the denominator.
![]()
Notice that there is a common gene in the numerator and the denominator,![]() The aught for this factor is
The aught for this factor is![]() This is the location of the removable aperture.
This is the location of the removable aperture.
Notice that there is a factor in the denominator that is non in the numerator,![]() The zero for this cistron is
The zero for this cistron is![]() The vertical asymptote is
The vertical asymptote is![]() Run across (Figure).
Run across (Figure).

The graph of this part will take the vertical asymptote at![]() simply at
simply at![]() the graph will have a pigsty.[/subconscious-respond]
the graph will have a pigsty.[/subconscious-respond]
Try It
Find the vertical asymptotes and removable discontinuities of the graph of![]()
[reveal-reply q="fs-id1165135203443″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135203443″]
Removable discontinuity at![]() Vertical asymptotes:
Vertical asymptotes:![]()
[/hidden-answer]
Identifying Horizontal Asymptotes of Rational Functions
While vertical asymptotes describe the behavior of a graph as the output gets very large or very minor, horizontal asymptotes help describe the beliefs of a graph every bit the input gets very large or very small. Remember that a polynomial'southward end beliefs will mirror that of the leading term. Too, a rational function's end behavior will mirror that of the ratio of the function that is the ratio of the leading terms.
There are 3 distinct outcomes when checking for horizontal asymptotes:
Case ane: If the degree of the denominator > degree of the numerator, in that location is a horizontal asymptote at![]()
![]()
In this example, the cease behavior is![]() This tells us that, as the inputs increase or decrease without jump, this function will behave similarly to the function
This tells us that, as the inputs increase or decrease without jump, this function will behave similarly to the function![]() and the outputs volition approach zero, resulting in a horizontal asymptote at
and the outputs volition approach zero, resulting in a horizontal asymptote at![]() See (Figure). Notation that this graph crosses the horizontal asymptote.
See (Figure). Notation that this graph crosses the horizontal asymptote.

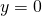 when
when
Case 2: If the degree of the denominator < degree of the numerator by i, we get a slant asymptote.
![]()
In this example, the stop behavior is![]() This tells us that as the inputs increment or decrease without bound, this function will conduct similarly to the function
This tells us that as the inputs increment or decrease without bound, this function will conduct similarly to the function![]() As the inputs grow large, the outputs will abound and not level off, then this graph has no horizontal asymptote. Nonetheless, the graph of
As the inputs grow large, the outputs will abound and not level off, then this graph has no horizontal asymptote. Nonetheless, the graph of![]() looks like a diagonal line, and since
looks like a diagonal line, and since![]() volition behave similarly to
volition behave similarly to![]() it will approach a line close to
it will approach a line close to![]() This line is a slant asymptote.
This line is a slant asymptote.
To discover the equation of the slant asymptote, separate![]() The quotient is
The quotient is![]() and the residue is 2. The slant asymptote is the graph of the line
and the residue is 2. The slant asymptote is the graph of the line![]() Come across (Figure).
Come across (Figure).
Case 3: If the degree of the denominator = degree of the numerator, there is a horizontal asymptote at![]() where
where![]() and
and![]() are the leading coefficients of
are the leading coefficients of![]() and
and![]() for
for![]()
![]()
In this case, the end behavior is![]() This tells united states of america that as the inputs grow large, this function will behave like the function
This tells united states of america that as the inputs grow large, this function will behave like the function![]() which is a horizontal line. Every bit
which is a horizontal line. Every bit![]() resulting in a horizontal asymptote at
resulting in a horizontal asymptote at![]() Come across (Figure). Annotation that this graph crosses the horizontal asymptote.
Come across (Figure). Annotation that this graph crosses the horizontal asymptote.
Notice that, while the graph of a rational function will never cross a vertical asymptote, the graph may or may non cantankerous a horizontal or slant asymptote. Also, although the graph of a rational function may take many vertical asymptotes, the graph will have at most i horizontal (or slant) asymptote.
It should be noted that, if the degree of the numerator is larger than the degree of the denominator by more than one, the end behavior of the graph will mimic the behavior of the reduced cease beliefs fraction. For example, if we had the function
![]()
with cease behavior
![]()
the end behavior of the graph would look similar to that of an even polynomial with a positive leading coefficient.
![]()
Horizontal Asymptotes of Rational Functions
The horizontal asymptote of a rational function can be determined past looking at the degrees of the numerator and denominator.
- Caste of numerator is less than degree of denominator: horizontal asymptote at

- Degree of numerator is greater than degree of denominator past one: no horizontal asymptote; camber asymptote.
- Degree of numerator is equal to degree of denominator: horizontal asymptote at ratio of leading coefficients.
Identifying Horizontal Asymptotes
In the sugar concentration trouble earlier, nosotros created the equation![]()
Find the horizontal asymptote and interpret it in context of the problem.
[reveal-respond q="fs-id1165137559522″]Show Solution[/reveal-reply]
[hidden-answer a="fs-id1165137559522″]
Both the numerator and denominator are linear (degree 1). Because the degrees are equal, at that place will be a horizontal asymptote at the ratio of the leading coefficients. In the numerator, the leading term is![]() with coefficient one. In the denominator, the leading term is
with coefficient one. In the denominator, the leading term is![]() with coefficient 10. The horizontal asymptote volition exist at the ratio of these values:
with coefficient 10. The horizontal asymptote volition exist at the ratio of these values:
![]()
This part will have a horizontal asymptote at![]()
This tells united states that every bit the values of t increase, the values of![]() will approach
will approach![]() In context, this means that, as more time goes by, the concentration of sugar in the tank will approach one-10th of a pound of saccharide per gallon of water or
In context, this means that, as more time goes by, the concentration of sugar in the tank will approach one-10th of a pound of saccharide per gallon of water or![]() pounds per gallon.[/hidden-answer]
pounds per gallon.[/hidden-answer]
Identifying Horizontal and Vertical Asymptotes
Find the horizontal and vertical asymptotes of the role
![]()
[reveal-reply q="fs-id1165137731893″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137731893″]
First, notation that this function has no common factors, so there are no potential removable discontinuities.
The function will have vertical asymptotes when the denominator is zero, causing the function to be undefined. The denominator will be cypher at![]() indicating vertical asymptotes at these values.
indicating vertical asymptotes at these values.
The numerator has degree two, while the denominator has degree 3. Since the degree of the denominator is greater than the degree of the numerator, the denominator will abound faster than the numerator, causing the outputs to tend towards zero equally the inputs go big, and so as![]() This function will take a horizontal asymptote at
This function will take a horizontal asymptote at![]() See (Effigy).
See (Effigy).

[/hidden-respond]
Try Information technology
Find the vertical and horizontal asymptotes of the function:
![]()
[reveal-reply q="fs-id1165137731188″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137731188″]
Vertical asymptotes at![]() and
and![]() horizontal asymptote at
horizontal asymptote at![]()
[/hidden-answer]
Intercepts of Rational Functions
A rational function will have a y-intercept at![]() , if the function is defined at cipher. A rational part will not accept a y-intercept if the function is not divers at goose egg.
, if the function is defined at cipher. A rational part will not accept a y-intercept if the function is not divers at goose egg.
Too, a rational function volition take x-intercepts at the inputs that cause the output to exist zero. Since a fraction is only equal to zero when the numerator is zilch, x-intercepts can only occur when the numerator of the rational function is equal to nix.
Finding the Intercepts of a Rational Function
Notice the intercepts of![]()
[reveal-answer q="fs-id1165134037668″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id1165134037668″]
We can find the y-intercept past evaluating the function at nothing

The x-intercepts will occur when the function is equal to zero:

The y-intercept is![]() the x-intercepts are
the x-intercepts are![]() and
and![]() See (Figure).
See (Figure).

[/hidden-answer]
Try It
Given the reciprocal squared function that is shifted right iii units and down 4 units, write this equally a rational function. Then, find the 10– and y-intercepts and the horizontal and vertical asymptotes.
[reveal-respond q="fs-id1165135183976″]Bear witness Solution[/reveal-reply]
[hidden-answer a="fs-id1165135183976″]
For the transformed reciprocal squared office, we find the rational course.![]()
Because the numerator is the same caste as the denominator we know that as![]() is the horizontal asymptote. Adjacent, nosotros set up the denominator equal to aught, and find that the vertical asymptote is
is the horizontal asymptote. Adjacent, nosotros set up the denominator equal to aught, and find that the vertical asymptote is![]() considering as
considering as![]() Nosotros then set up the numerator equal to 0 and observe the ten-intercepts are at
Nosotros then set up the numerator equal to 0 and observe the ten-intercepts are at![]() and
and![]() Finally, nosotros evaluate the function at 0 and find the y-intercept to be at
Finally, nosotros evaluate the function at 0 and find the y-intercept to be at![]()
[/subconscious-answer]
Graphing Rational Functions
In (Effigy), nosotros see that the numerator of a rational function reveals the x-intercepts of the graph, whereas the denominator reveals the vertical asymptotes of the graph. As with polynomials, factors of the numerator may have integer powers greater than one. Fortunately, the effect on the shape of the graph at those intercepts is the aforementioned as we saw with polynomials.
The vertical asymptotes associated with the factors of the denominator volition mirror one of the 2 toolkit reciprocal functions. When the degree of the cistron in the denominator is odd, the distinguishing characteristic is that on i side of the vertical asymptote the graph heads towards positive infinity, and on the other side the graph heads towards negative infinity. See (Effigy).

When the degree of the cistron in the denominator is fifty-fifty, the distinguishing feature is that the graph either heads toward positive infinity on both sides of the vertical asymptote or heads toward negative infinity on both sides. See (Figure).

For example, the graph of![]() is shown in (Figure).
is shown in (Figure).

How To
Given a rational function, sketch a graph.
- Evaluate the function at 0 to observe the y-intercept.
- Factor the numerator and denominator.
- For factors in the numerator not common to the denominator, determine where each factor of the numerator is goose egg to find the x-intercepts.
- Find the multiplicities of the x-intercepts to determine the beliefs of the graph at those points.
- For factors in the denominator, note the multiplicities of the zeros to determine the local beliefs. For those factors not common to the numerator, find the vertical asymptotes past setting those factors equal to zip then solve.
- For factors in the denominator mutual to factors in the numerator, find the removable discontinuities by setting those factors equal to 0 and and so solve.
- Compare the degrees of the numerator and the denominator to determine the horizontal or slant asymptotes.
- Sketch the graph.
Graphing a Rational Function
Sketch a graph of![]()
[reveal-answer q="fs-id1165137413858″]Show Solution[/reveal-reply]
[hidden-respond a="fs-id1165137413858″]
Nosotros can start by noting that the role is already factored, saving the states a pace.
Next, nosotros volition find the intercepts. Evaluating the function at zero gives the y-intercept:
![]()
To find the 10-intercepts, we determine when the numerator of the part is zero. Setting each factor equal to nothing, we find x-intercepts at![]() and
and![]() At each, the beliefs will be linear (multiplicity i), with the graph passing through the intercept.
At each, the beliefs will be linear (multiplicity i), with the graph passing through the intercept.
Nosotros have a y-intercept at![]() and x-intercepts at
and x-intercepts at![]() and
and![]()
To notice the vertical asymptotes, we determine when the denominator is equal to zero. This occurs when![]() and when
and when![]() giving us vertical asymptotes at
giving us vertical asymptotes at![]() and
and![]()
There are no common factors in the numerator and denominator. This means in that location are no removable discontinuities.
Finally, the caste of denominator is larger than the degree of the numerator, telling united states this graph has a horizontal asymptote at![]()
To sketch the graph, we might start by plotting the three intercepts. Since the graph has no ten-intercepts between the vertical asymptotes, and the y-intercept is positive, we know the function must remain positive betwixt the asymptotes, letting us fill in the middle portion of the graph as shown in (Figure).

The factor associated with the vertical asymptote at![]() was squared, so we know the behavior will be the aforementioned on both sides of the asymptote. The graph heads toward positive infinity as the inputs arroyo the asymptote on the right, and then the graph will head toward positive infinity on the left likewise.
was squared, so we know the behavior will be the aforementioned on both sides of the asymptote. The graph heads toward positive infinity as the inputs arroyo the asymptote on the right, and then the graph will head toward positive infinity on the left likewise.
For the vertical asymptote at![]() the factor was not squared, so the graph will take opposite beliefs on either side of the asymptote. Run across (Effigy). After passing through the x-intercepts, the graph volition then level off toward an output of zero, every bit indicated by the horizontal asymptote.
the factor was not squared, so the graph will take opposite beliefs on either side of the asymptote. Run across (Effigy). After passing through the x-intercepts, the graph volition then level off toward an output of zero, every bit indicated by the horizontal asymptote.

[/subconscious-answer]
Attempt It
Given the part![]() use the characteristics of polynomials and rational functions to depict its behavior and sketch the office.
use the characteristics of polynomials and rational functions to depict its behavior and sketch the office.
[reveal-answer q="fs-id1165137454284″]Show Solution[/reveal-answer]
[subconscious-respond a="fs-id1165137454284″]
Horizontal asymptote at![]() Vertical asymptotes at
Vertical asymptotes at![]() y-intercept at
y-intercept at![]()
x-intercepts at![]() [latex]\left(–ii,0\correct)\,[/latex]is a zero with multiplicity 2, and the graph bounces off the 10-axis at this betoken.
[latex]\left(–ii,0\correct)\,[/latex]is a zero with multiplicity 2, and the graph bounces off the 10-axis at this betoken.![]() is a unmarried nix and the graph crosses the axis at this point.
is a unmarried nix and the graph crosses the axis at this point.

[/hidden-answer]
Writing Rational Functions
Now that nosotros have analyzed the equations for rational functions and how they relate to a graph of the function, we can use information given past a graph to write the function. A rational function written in factored grade will have an x-intercept where each factor of the numerator is equal to cypher. (An exception occurs in the case of a removable discontinuity.) As a result, we can form a numerator of a function whose graph volition pass through a set of ten-intercepts past introducing a corresponding set of factors. Likewise, because the function volition have a vertical asymptote where each cistron of the denominator is equal to zero, we can form a denominator that will produce the vertical asymptotes by introducing a respective gear up of factors.
How To
Given a graph of a rational function, write the function.
- Make up one's mind the factors of the numerator. Examine the behavior of the graph at the 10-intercepts to determine the zeroes and their multiplicities. (This is easy to practise when finding the "simplest" part with small multiplicities—such as 1 or iii—just may exist difficult for larger multiplicities—such as v or 7, for case.)
- Determine the factors of the denominator. Examine the behavior on both sides of each vertical asymptote to determine the factors and their powers.
- Apply any clear signal on the graph to find the stretch gene.
Writing a Rational Function from Intercepts and Asymptotes
Write an equation for the rational office shown in (Figure).

[reveal-answer q="fs-id1165137767514″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137767514″]
The graph appears to have x-intercepts at![]() and
and![]() At both, the graph passes through the intercept, suggesting linear factors. The graph has ii vertical asymptotes. The i at
At both, the graph passes through the intercept, suggesting linear factors. The graph has ii vertical asymptotes. The i at![]() seems to exhibit the basic behavior similar to
seems to exhibit the basic behavior similar to![]() with the graph heading toward positive infinity on i side and heading toward negative infinity on the other. The asymptote at
with the graph heading toward positive infinity on i side and heading toward negative infinity on the other. The asymptote at![]() is exhibiting a behavior similar to
is exhibiting a behavior similar to![]() with the graph heading toward negative infinity on both sides of the asymptote. See (Figure).
with the graph heading toward negative infinity on both sides of the asymptote. See (Figure).

We can utilize this information to write a office of the grade
![]()
To find the stretch factor, we can use another clear signal on the graph, such as the y-intercept![]()
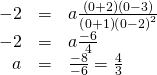
This gives the states a final function of![]() [/hidden-reply]
[/hidden-reply]
Key Equations
| Rational Function | |
Key Concepts
![]()
Meet (Figure).
Department Exercises
Verbal
What is the fundamental difference in the algebraic representation of a polynomial function and a rational role?
[reveal-respond q="fs-id1165137848972″]Show Solution[/reveal-respond]
[hidden-answer a="fs-id1165137848972″]
The rational function will be represented by a quotient of polynomial functions.
[/hidden-answer]
What is the fundamental difference in the graphs of polynomial functions and rational functions?
If the graph of a rational function has a removable discontinuity, what must be true of the functional rule?
[reveal-reply q="fs-id1165135518222″]Show Solution[/reveal-reply]
[subconscious-respond a="fs-id1165135518222″]
The numerator and denominator must have a common gene.
[/subconscious-answer]
Can a graph of a rational role take no vertical asymptote? If so, how?
Can a graph of a rational function have no x-intercepts? If so, how?
[reveal-answer q="fs-id1165135393372″]Show Solution[/reveal-reply]
[hidden-respond a="fs-id1165135393372″]
Yes. The numerator of the formula of the functions would take only circuitous roots and/or factors mutual to both the numerator and denominator.
[/hidden-answer]
Algebraic
For the following exercises, notice the domain of the rational functions.
![]()
![]()
[reveal-reply q="fs-id1165134122925″]Show Solution[/reveal-answer]
[hidden-respond a="fs-id1165134122925″]
![]()
[/hidden-respond]
![]()
![]()
[reveal-answer q="fs-id1165137619914″]Show Solution[/reveal-answer]
[subconscious-reply a="fs-id1165137619914″]
![]()
[/subconscious-reply]
For the following exercises, find the domain, vertical asymptotes, and horizontal asymptotes of the functions.
![]()
![]()
[reveal-reply q="fs-id1165135181414″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135181414″]
V.A. at![]() H.A. at
H.A. at![]() Domain is all reals
Domain is all reals![]()
[/hidden-respond]
![]()
![]()
[reveal-reply q="fs-id1165135412875″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135412875″]
V.A. at![]() H.A. at
H.A. at![]() Domain is all reals
Domain is all reals![]()
[/subconscious-answer]
![]()
![]()
[reveal-answer q="fs-id1165135443834″]Prove Solution[/reveal-reply]
[hidden-answer a="fs-id1165135443834″]
Five.A. at![]() H.A. at
H.A. at![]() Domain is all reals
Domain is all reals![]()
[/hidden-answer]
![]()
![]()
[reveal-respond q="fs-id1165135192050″]Evidence Solution[/reveal-answer]
[subconscious-answer a="fs-id1165135192050″]
5.A. at![]() H.A. at
H.A. at![]() Domain is all reals
Domain is all reals![]()
[/hidden-answer]
![]()
![]()
[reveal-respond q="fs-id1165137737944″]Show Solution[/reveal-respond]
[subconscious-answer a="fs-id1165137737944″]
V.A. at![]() H.A. at
H.A. at![]() Domain is all reals
Domain is all reals![]()
[/hidden-answer]
For the following exercises, find the 10– and y-intercepts for the functions.
![]()
![]()
[reveal-answer q="fs-id1165137727815″]Show Solution[/reveal-reply]
[hidden-respond a="fs-id1165137727815″]
none
[/hidden-answer]
![]()
![]()
[reveal-answer q="fs-id1165137762062″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137762062″]
![]()
[/subconscious-answer]
![]()
For the following exercises, describe the local and end behavior of the functions.
![]()
[reveal-answer q="fs-id1165137527922″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137527922″]
Local behavior:![]()
Cease beliefs:![]()
[/hidden-answer]
![]()
![]()
[reveal-reply q="fs-id1165137793829″]Prove Solution[/reveal-answer]
[subconscious-respond a="fs-id1165137793829″]
Local beliefs:![]() End behavior:
End behavior:![]()
[/hidden-answer]
![]()
![]()
[reveal-answer q="fs-id1165134073889″]Bear witness Solution[/reveal-respond]
[hidden-answer a="fs-id1165134073889″]
Local behavior:![]() [latex]f\left(ten\right)\to -\infty ,x\to {\frac{5}{2}}^{-},f\left(x\right)\to \infty ,10\to {\frac{5}{2}}^{+},f\left(10\right)\to -\infty [/latex]
[latex]f\left(ten\right)\to -\infty ,x\to {\frac{5}{2}}^{-},f\left(x\right)\to \infty ,10\to {\frac{5}{2}}^{+},f\left(10\right)\to -\infty [/latex]
End behavior: ![]()
[/hidden-respond]
For the post-obit exercises, find the slant asymptote of the functions.
![]()
![]()
[reveal-respond q="fs-id1165137803153″]Bear witness Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137803153″]
![]()
[/hidden-answer]
![]()
![]()
[reveal-answer q="fs-id1165135152039″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135152039″]
![]()
[/hidden-respond]
![]()
Graphical
For the following exercises, use the given transformation to graph the function. Note the vertical and horizontal asymptotes.
The reciprocal function shifted up ii units.
[reveal-answer q="fs-id1165137749319″]Testify Solution[/reveal-answer]
[hidden-reply a="fs-id1165137749319″]
![]()
 [/hidden-answer]
[/hidden-answer]
The reciprocal function shifted down one unit and left 3 units.
The reciprocal squared part shifted to the right 2 units.
[reveal-answer q="fs-id1165137673413″]Show Solution[/reveal-answer]
[hidden-respond a="fs-id1165137673413″]
![]()
 [/hidden-answer]
[/hidden-answer]
The reciprocal squared function shifted down 2 units and right 1 unit.
For the post-obit exercises, observe the horizontal intercepts, the vertical intercept, the vertical asymptotes, and the horizontal or camber asymptote of the functions. Employ that data to sketch a graph.
![]()
[reveal-answer q="fs-id1165137832470″]Evidence Solution[/reveal-answer]
[hidden-respond a="fs-id1165137832470″]
![]()

[/hidden-respond]
![]()
![]()
[reveal-respond q="fs-id1165135533080″]Prove Solution[/reveal-answer]
[hidden-reply a="fs-id1165135533080″]
![]()

[/hidden-answer]
![]()
![]()
[reveal-respond q="fs-id1165135195965″]Evidence Solution[/reveal-answer]
[hidden-answer a="fs-id1165135195965″]
![]()

[/subconscious-answer]
![]()
![]()
[reveal-answer q="fs-id1165137635057″]Testify Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137635057″]
![]()

[/subconscious-answer]
![]()
![]()
[reveal-respond q="fs-id1165135208397″]Evidence Solution[/reveal-answer]
[subconscious-answer a="fs-id1165135208397″]
![]()

[/hidden-answer]
![]()
![]()
[reveal-answer q="fs-id1165135264668″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135264668″]
![]()

[/hidden-answer]
![]()
For the post-obit exercises, write an equation for a rational function with the given characteristics.
Vertical asymptotes at![]() and
and![]() x-intercepts at
x-intercepts at![]() and
and![]() y-intercept at
y-intercept at![]()
[reveal-reply q="fs-id1165137766733″]Show Solution[/reveal-respond]
[hidden-answer a="fs-id1165137766733″]
![]()
[/hidden-answer]
Vertical asymptotes at![]() and
and![]() x-intercepts at
x-intercepts at![]() and
and![]() Horizontal asymptote at
Horizontal asymptote at![]()
[reveal-answer q="fs-id1165134328215″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id1165134328215″]
![]()
[/hidden-answer]
Vertical asymptote at![]() Double zero at
Double zero at![]() y-intercept at
y-intercept at![]()
[reveal-answer q="fs-id1165137832215″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137832215″]
![]()
[/hidden-answer]
For the following exercises, use the graphs to write an equation for the function.

[reveal-answer q="fs-id1165131990659″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165131990659″]
![]()
[/hidden-answer]


[reveal-answer q="fs-id1165137936729″]Show Solution[/reveal-respond]
[hidden-respond a="fs-id1165137936729″]
![]()
[/hidden-answer]


[reveal-answer q="fs-id1165137414994″]Show Solution[/reveal-respond]
[subconscious-answer a="fs-id1165137414994″]
![]()
[/subconscious-answer]


[reveal-answer q="fs-id1165137732796″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137732796″]
![]()
[/subconscious-answer]

Numeric
For the following exercises, make tables to prove the behavior of the role almost the vertical asymptote and reflecting the horizontal asymptote
![]()
[reveal-answer q="fs-id1165137653644″]Testify Solution[/reveal-reply]
[hidden-respond a="fs-id1165137653644″]
| | ii.01 | 2.001 | 2.0001 | one.99 | 1.999 |
| | 100 | 1,000 | 10,000 | –100 | –1,000 |
| | 10 | 100 | 1,000 | 10,000 | 100,000 |
|---|---|---|---|---|---|
| | .125 | .0102 | .001 | .0001 | .00001 |
Vertical asymptote![]() Horizontal asymptote
Horizontal asymptote![]()
[/hidden-answer]
![]()
![]()
[reveal-answer q="fs-id1165135501151″]Testify Solution[/reveal-answer]
[hidden-answer a="fs-id1165135501151″]
| | –4.1 | –4.01 | –iv.001 | –3.99 | –3.999 |
| | 82 | 802 | eight,002 | –798 | –7998 |
| | 10 | 100 | 1,000 | ten,000 | 100,000 |
| | ane.4286 | one.9331 | 1.992 | one.9992 | ane.999992 |
Vertical asymptote![]() Horizontal asymptote
Horizontal asymptote![]()
[/subconscious-answer]
![]()
![]()
[reveal-answer q="fs-id1165137779967″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165137779967″]
| | –.9 | –.99 | –.999 | –1.1 | –1.01 |
| | 81 | 9,801 | 998,001 | 121 | 10,201 |
| | 10 | 100 | i,000 | x,000 | 100,000 |
| | .82645 | .9803 | .998 | .9998 |
Vertical asymptote![]() Horizontal asymptote
Horizontal asymptote![]()
[/hidden-reply]
Technology
For the following exercises, use a calculator to graph![]() Utilise the graph to solve
Utilise the graph to solve![]()
![]()
![]()
[reveal-reply q="fs-id1165135551843″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165135551843″]
![]()
 [/hidden-answer]
[/hidden-answer]
![]()
![]()
[reveal-reply q="fs-id1165137742755″]Show Solution[/reveal-respond]
[hidden-answer a="fs-id1165137742755″]
![]()
 [/hidden-answer]
[/hidden-answer]
![]()
Extensions
For the post-obit exercises, identify the removable discontinuity.
![]()
[reveal-answer q="fs-id1165135195746″]Testify Solution[/reveal-answer]
[hidden-reply a="fs-id1165135195746″]
![]()
[/subconscious-answer]
![]()
![]()
[reveal-respond q="fs-id1165134261663″]Testify Solution[/reveal-reply]
[hidden-answer a="fs-id1165134261663″]
![]()
[/hidden-respond]
![]()
![]()
[reveal-answer q="fs-id1165137762314″]Evidence Solution[/reveal-answer]
[hidden-answer a="fs-id1165137762314″]
![]()
[/hidden-answer]
Real-Earth Applications
For the following exercises, express a rational office that describes the state of affairs.
A big mixing tank currently contains 200 gallons of h2o, into which 10 pounds of sugar take been mixed. A tap volition open, pouring 10 gallons of water per minute into the tank at the aforementioned fourth dimension carbohydrate is poured into the tank at a rate of 3 pounds per minute. Detect the concentration (pounds per gallon) of sugar in the tank after![]() minutes.
minutes.
A large mixing tank currently contains 300 gallons of water, into which viii pounds of sugar have been mixed. A tap will open, pouring twenty gallons of water per minute into the tank at the same fourth dimension saccharide is poured into the tank at a charge per unit of 2 pounds per minute. Discover the concentration (pounds per gallon) of sugar in the tank after![]() minutes.
minutes.
[reveal-respond q="fs-id1165135581177″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id1165135581177″]
![]()
[/subconscious-answer]
For the post-obit exercises, utilize the given rational function to reply the question.
The concentration![]() of a drug in a patient's bloodstream
of a drug in a patient's bloodstream ![]() hours later on injection is given by
hours later on injection is given by![]() Utilise a estimator to approximate the fourth dimension when the concentration is highest.
Utilise a estimator to approximate the fourth dimension when the concentration is highest.
[reveal-answer q="fs-id1165135368500″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id1165135368500″]
Subsequently about 6.12 hours.
[/hidden-answer]
For the following exercises, construct a rational role that will help solve the problem. And so, use a figurer to reply the question.
An open box with a foursquare base is to take a volume of 108 cubic inches. Observe the dimensions of the box that will have minimum surface expanse. Let![]() = length of the side of the base.
= length of the side of the base.
A rectangular box with a square base of operations is to have a volume of xx cubic anxiety. The textile for the base costs 30 cents/ square foot. The material for the sides costs 10 cents/square foot. The cloth for the pinnacle costs 20 cents/square foot. Determine the dimensions that volition yield minimum cost. Permit![]() = length of the side of the base.
= length of the side of the base.
[reveal-answer q="fs-id1165137940592″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id1165137940592″]
![]() two by 2 by five feet.
two by 2 by five feet.
[/hidden-answer]
A correct round cylinder has volume of 100 cubic inches. Find the radius and height that will yield minimum surface area. Let![]() = radius.
= radius.
A right circular cylinder with no elevation has a volume of 50 cubic meters. Find the radius that volition yield minimum expanse. Allow![]() = radius.
= radius.
[reveal-answer q="fs-id1165137436431″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1165137436431″]
![]() Radius = 2.52 meters.
Radius = 2.52 meters.
[/hidden-answer]
A right circular cylinder is to have a volume of forty cubic inches. Information technology costs 4 cents/square inch to construct the tiptop and bottom and 1 cent/foursquare inch to construct the rest of the cylinder. Observe the radius to yield minimum cost. Let![]() = radius.
= radius.
Source: https://opentextbc.ca/algebratrigonometryopenstax/chapter/rational-functions/
Posted by: johnsonsawn1984.blogspot.com

0 Response to "how to find y intercept of a rational function"
Post a Comment